In addition to solving limit problems numerically (with your calculator) and symbolically (with algebra), you should be able to solve limit and continuity problems visually. The following practice questions will test your skills.
Use the following figure to answer the practice problems.
PRACTICE QUESTIONS
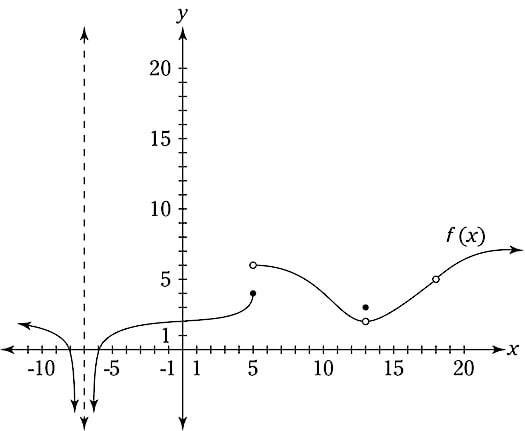
- f(5) = ?
- f(18) = ?
- List the x coordinates of all discontinuities of f, state whether the discontinuities are removable or nonremovable, and give the type of discontinuity—hole, jump, or infinite.
ANSWERS AND EXPLANATIONS
- f(5) = 4, the height of the solid dot at x = 5.
- f(18) is undefined because f has no y value corresponding to the x value of 18.
- At x = –7, the vertical asymptote, there is a nonremovable, infinite discontinuity. At x = 5, there’s a nonremovable, jump discontinuity. At x = 13 and x = 18, there are holes which are removable discontinuities.
Though infinitely small, these are nevertheless discontinuities. They’re “removable” discontinuities because you can “fix” the function by plugging the holes.